Question

Compare the following: i. The buoyant force exerted by the water on the 3 kg cube to the buoyant force exerted on the 1 kg cube. ii. The tension in the string holding the 3 kg cube to the tension in the string holding the 1 kg cube. iii. The pressure exerted on the bottom surface of the 3 kg cube by the water to the pressure on the bottom surface of the 1 kg cube.

Answer 1

The buoyant force on the cubes will be the same, the tension in the strings holding the cubes will be the same, but the pressure exerted on the bottom surface of the 3 kg cube will be greater than the pressure on the bottom surface of the 1 kg cube.

Step-by-step explanation:

The buoyant force exerted by the water on the 3 kg cube can be compared to the buoyant force exerted on the 1 kg cube. The buoyant force experienced by an object is equal to the weight of the fluid it displaces. Since both cubes are completely submerged in water, they displace the same volume of water. Therefore, the buoyant force on both cubes will be the same.

The tension in the string holding the 3 kg cube can be compared to the tension in the string holding the 1 kg cube. The tension in a string is equal throughout its length when the string is in equilibrium. Since both strings are attached to the same object, they will be under the same tension.

The pressure exerted on the bottom surface of the 3 kg cube by the water compared to the pressure on the bottom surface of the 1 kg cube can be analyzed using the equation of pressure: pressure = force/area. As the cubes have different masses and volumes, their weights will be different but the area of their bottom surfaces will be the same. Therefore, the pressure exerted by the water on the bottom surface of the 3 kg cube will be greater than the pressure on the bottom surface of the 1 kg cube.

Answer 2

i) B₃> B₁, ii) submerged body T>0, body in equilibrium or floats T=0,

iii) the same volume P₃ = P₁, different volume P₃> P₁

Step-by-step explanation:

i) The thrust force is equal to the weight of the desalted liquid

B = W = ρ g
`V_(liquid)`

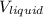
with this expression let's analyze the situation, we have two possibilities

* The two cubes have the same volume (bodies with different density) in this case The thrust on the heavier cube is greater

cube m = 3 kg B₃ = 3 9.8

B₃ = 29.4 N

cube m = 1 kg B₁ = 1 9.8

B₁ = 9.8 n

B₃> B₁

* The two cubes have different volume (same density), in this case the body with greater mass must have more volume

ρ = m / V

m = ρ V

because we have assumed that the density is the same

cube m = 3 kg B₃ = ρ g V₃

cube m = 1 kg B₁ = ρ g V₁

for this second possibility also B₃> B₁

ii) the tension in the rope that supports each cube

let's use the equilibrium condition

T + B - W = 0

T = W -B

in this case we have several possibilities, depending on whether the cube can float or sink in the liquid which changed the thrust

a) submerged body sinks in the liquid therefore the weight of the body is greater than the thrust of the liquid

T> 0

b) body in equilibrium in the liquid, in this case the thrust and weight are equal

T = 0

c) the body floats in the liquid, in this case only part of the volume of the body is submerged and this part is the one that creates a thrust (B ') less than et in equilibrium with the weight

T = W- B '

T = 0

We can see that the only case where the tension is different from zero is when the body sinks, for this possibility, as the thrust of the heavier body is greater

cube m = 3kg T₃ = W₃ -B₃

cube m = 1 kg T₁ = W₁ - B₁

For this case, the density of the body must be known to calculate especially the thrust in each case, if the two bodies have the same density

T₃> T₁

iii) The pressure on the lower part of the cube, there are several cases

* The two cubes with the same volume, in this case the height of the cubes is the same

P = ρ g h

the pressure for both cubes is the same

P₃ = P₁

* the two have different volume, we assume that h₃> h₁

therefore P₃> P₁