Question

[NOTE: There are two ways to do this problem. The first is the quotient rule. The second is much easier and does not use the quotient rule.]

Answer 1

a. 1/3

b. 0

EXPLANATION :

From the problem, we have the function :

`f(x)=(x^2+6x+8)/(3x+12)`

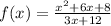
Factor the numerator and the denominator :

`\begin{gathered} f(x)=((x+4)(x+2))/(3(x+4)) \\ f(x)=(x+2)/(3) \end{gathered}`

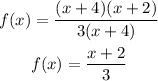
a. Getting the first derivative :

`\begin{gathered} f^(\prime)(x)=(1)/(3)(1) \\ f^(\prime)(x)=(1)/(3) \end{gathered}`

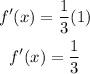
So what ever the value of x, the derivative is always 1/3

The answer is 1/3

b. f''(5), the derivative of any constant is always 0.

Since the first derivative is a constant, therefore, the second derivative is 0.