Question

Two supplementry angles are in the ratio of 5:7 . find the measure of the angles

Answer 1

75 degrees and 105 degrees

Explanation:

Supplementary angles are those angles that sum up to 180 degrees

So angle A + B = 180 or B = 180 - A

then A/B = 5/7

7A = 5B

7A = 5(180 - A)

7A = 900 - 5A

12A = 900

A = 75

then B = 180 - A = 180 - 75 = 105

Answer 2

Let the the two given supplementary angles be A & B respectively. Ratio of angle A to the ratio of angle B is 5/7. And we've been asked to find out the measure of angle A & B.

As we know that supplementary angle are the ones which have the sum of angles and tend to 180°. Thus we can write by forming equation that,

`:\implies\rm{a + b = 180 \: ...(1)}`

`:\implies\rm{b = 180 - a \: ...(2)}`

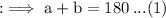
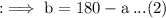
Now as per the given ratio we can write,

`:\implies\rm{ (a)/(b) = (5)/(7) }`

`:\implies\rm{7a = 5b}`

`:\implies\rm{7a = 5(180 - a) \: ...(from \: 2)}`

`:\implies\rm{7a = 900 - 5a}`

`:\implies\rm{7a + 5a = 900}`

`:\implies\rm{12a = 900}`

`:\implies\rm{a = (900)/(12) }`

`:\implies\rm{a = 75}`

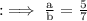
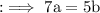
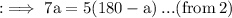
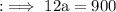
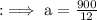
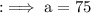
Now by equation (2) we can write,

`:\implies\rm{b = 180 - a \: ...(from \: 2)}`

`:\implies\rm{b = 180 - 75}`

`:\implies\rm{b = 105}`

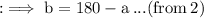
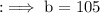
• The measure of angles are 75° and 105°.