Question

What is the equation of the quadratic graph with a focus of (2, − 2) and a directrix of y = 0?

Answer 1

f=(h,k+p) = (2,-2)
then h = 2
and -2= k + p
Directrix = 0 = k - p

Solve these 2 equations and 2 unknowns yields k=-1 and p=-1
The equation is therefore,
y=(1/4p)(x - 2)^2 -1

Answer 2

The equation of the parabola is
`(x-2)^2=-4(y+1)`.

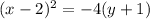Explanation:

The standard form of the parabola is,

`(x-h)^2=4p(y-k)`

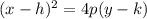
Where, (h,k+p) is focus and directrix is y=k-p

It is given that the focus of (2,-2) and a directrix of y = 0

`(h,k+p)=(2,-2)`

`h=2`

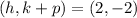
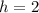
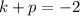`k+p= -2` ... (1)

Since directrix is y=0,

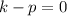`k-p=0` ... (2)

Add equation 1 and 2.

`2k=-2`

`k=-1`

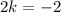
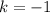
Put this value in equation 2.

`-1-p=0`

`p=-1`

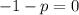
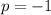
Now we have p= -1, k= -1and h=2.

The equation of the parabola is,

`(x-2)^2=4(-1)(y+1)`

`(x-2)^2=-4(y+1)`

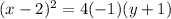
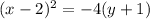
Therefore the equation of the parabola is
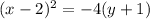`(x-2)^2=-4(y+1)`.