Question

Please help me work through this if you can, thank you!

Answer 1

Given:

`C=(x^4)/(4)-(4)/(3)x^3-(35)/(2)x^2+150x`

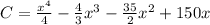
Let's solve for the following:

• (a). Find C'(x).

Here, we are to find the derivative of C(x).

Apply the sum rule:

`\begin{gathered} C^(\prime)=(d)/(dx)((x^4)/(4))+(d)/(dx)(-(4)/(3)x^3)+(d)/(dx)(-(35)/(2)x^2)+(d)/(dx)(150x) \\ \\ C^(\prime)=x^3-4x^2-35x+150 \end{gathered}`

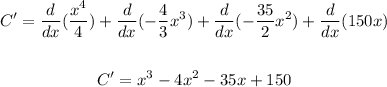
• (b). The critical numbers of C(x).

The critical numbers will be the points where the graph changes direction.

Using the derivative, let's solve for x.

`x^3-4x^2-35x+150=0`

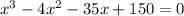
Factor the left side using the rational root test:

`(x-5)(x^2+x-30)=0`

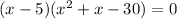
Now factor using the AC method:

`\begin{gathered} (x-5)((x-5)(x+6))=0 \\ \\ (x-5)(x-5)(x+6)=0 \end{gathered}`

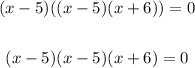
Equate each factor to zero and solve for x:

`\begin{gathered} x-5=0 \\ \text{ Add 5 to both sides:} \\ x-5+5=0+5 \\ x=5 \\ \\ \\ x-5=0 \\ x-5+5=0+5 \\ x=5 \\ \\ \\ x+6=0 \\ x+6-6=0-6 \\ x=-6 \end{gathered}`

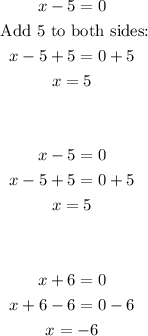
Therefore, the critical numbers of C(x) are:

x = -6, 5

• (C). Increasing interval.

Use the critical points to find the increasing and decreasing intervals.

Using interval notation, the increasing interval is:

`(-6,5)\cup(5,\infty)`

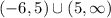
• D. Decreasing interval:

Using interval notation, the decreasing interval is:

`(-\infty,-6)`

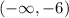
ANSWER:

(a). C'(x) = x³ - 4x² - 35x + 150

(b). x = -6, 5

(c). Increasing: (-6, 5) U (5,∞)

Decreasing: (-∞, -6)