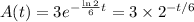Question

Oil is pumped continuously from a well at a rate proportional to the amount of oil left in the well. Initially there were 3 million barrels of oil in the well; six years later 1,500,000 barrels remain.

Answer 1

If
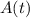
`A(t)` is the amount of oil in the well (let's say in millions of barrels) at time
`image`

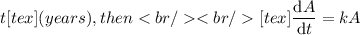
for some negative value of
`k`. You're told that the well started off with 3 million barrels, so
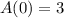
`A(0)=3`, and that after 6 years, there are 1.5 million barrels, so
`A(6)=1.5`.

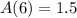
Solve the differential equation above:


`(\mathrm dA)/(\mathrm dt)=kA\implies\frac{\mathrm dA}A=k\,\mathrm dt`

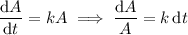
Integrate both sides.


`\displaystyle\int\frac{\mathrm dA}A=k\int\mathrm dt`

`\ln|A|=kt+C`

`A=e^(kt+C)`

`A=Ce^(kt)`

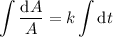
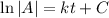
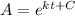
Given that
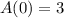
`A(0)=3` and
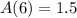
`A(6)=1.5`, you have


`\begin{cases}3=Ce^(0k)\\1.5=Ce^(6k)\end{cases}\implies C=3,\,k=-\frac{\ln2}6`

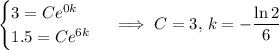
So the amount of oil in the well is given by the function


`A(t)=3e^{-\frac{\ln2}6t}=3*2^(-t/6)`