Question

You forget the last two digits of your password for a website. what is the probability that you randomly choose the correct digits? write the probability as a percent. this is %.

Answer 1

There are 100 possible choices, which are 00, 01, 02, 03... 99. Because there are 100 possible choices, the probability of guessing the correct digits is 1%.

Answer 2

The probability is
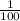`(1)/(100)`. In percentage form, it is 1%

Explanation:

The concern is the last two digits. First, we need to find the number of possible combinations for the missing digits.

First Digit: can be any number from 0 to 9, so there are 10 possible numbers

Second Digit: can also be any number from 0 to 9, so there are 10 possible numbers

So, the total possibility is 10 × 10 = 100

The probability then, of choosing the correct digits is 1 in a 100 possibilities, which implies that the probability is
`(1)/(100)`

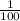
In percent form, % refers to one-hundredth, that is
`(1)/(100)`

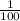
So, the probability is 1%