Question

The radius r of a circle can be written as a function of the area A with the following equation: What is the domain of this function? Explain why it makes sense in this context.

Answer 1

[0,infinity) because you can not take the radius of a negative mumber.

Answer 2

Hence, the domain of the function is:

[0,∞)

Explanation:

We know that area of circle is given by the function:

`A=\pi r^2`

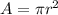
The radius r of a circle can be written as a function of the area A with the following equation:

Now we can represent r in terms of A as:

`r^2=(A)/(\pi)\\\\r=\sqrt{(A)/(\pi)}`

Now as we know that for the square root term to exist:

`\sqrt{(A)/(\pi)}\geq0`

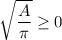
i.e.
`A\geq0`

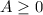
A=0 represents a point circle since it's area is zero.

Hence, the domain of the function is:

[0,∞)