Question

Uppose y(t)=9e−5t

y
(
t
)
=
9
e
−
5
t
is a solution of the initial value problem y′+ky=0
y
′
+
k
y
=
0
, y(0)=y0
y
(
0
)
=
y
0
. What are the constants k
k
and y

Answer 1

If
`y(t)=9e^(-5t)` satisfies the ODE
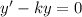
`y'-ky=0`, then


`y'(t)=-45e^(-5t)`

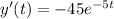
and substituting into the ODE gives


`-45e^(-5t)-9ke^(-5t)=0\implies k=-5`

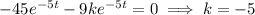
so that the ODE is
`y'+5y=0`.

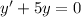
I'm assuming that you're also supposed to determine the initial value
`y_0` given this ODE. You have


`y'+5y=0\implies e^(5t)y'+5e^(5t)y=0\implies(e^(5t)y)'=0\implies e^(5t)y=C\implies y=Ce^(-5t)`

Given
`y(0)=y_0`, you have


`y_0=C`

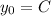
but since you already know that
`y=9e^(-5t)`, it follows that
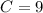
`C=9`, and in turn that
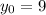
`y_0=9`.