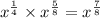Question

Multiply x to the 1 fourth power times x to the 5 eighths power.

Answer 1

x ^(1/4) * x^(5/8)
The rule is keep the base and add the powers.
Change 1/4 to a denominator of 8. 1/4 = 2/8
x ^(2/8 + 5/8)
x^ (7/8)

Answer 2

`x^{(1)/(4)}* x^{(5)/(8)}=x^{(7)/(8)}`

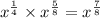
Explanation:

Given : Expression x to the 1 fourth power times x to the 5 eighths power.

To find : Multiply the expression?

Solution :

Step 1 - Write the expression,

x to the 1 fourth power -
`x^{(1)/(4)}`

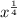
x to the 5 eighths power -
`x^{(5)/(8)}`

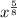
Expression -
`x^{(1)/(4)}* x^{(5)/(8)}`

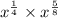
Step 2 - In multiply if bases are same power get added,

`=x^{(1)/(4)+(5)/(8)}`

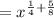
Step 3 - Solve,

`=x^{(2+5)/(8)}`

`=x^{(7)/(8)}`

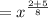
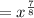
Therefore,
`x^{(1)/(4)}* x^{(5)/(8)}=x^{(7)/(8)}`