Answer:
Option (c) is correct.
Center of circle is at (6, -3)
Explanation:
Given : The equation of circle is
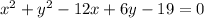
We have to find the center of given circle and choose from the given options.
The standard equation of circle with center (h,k) and radius r is written as
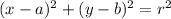
Consider the given equation
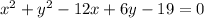
Adding 19 both side, we have,

Group x and y terms together, we have,
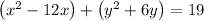
Add both side 36 to make x a perfect square term,
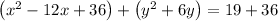
Using identity
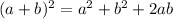 , we have,
, we have,
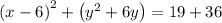
Similarly, for y , adding 9 both side,
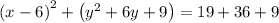
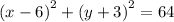
rewriting in standard form , we have,
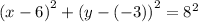
Thus, Center of circle is at (6, -3)
Option (c) is correct.