Question

The 2 triangles shown in the figure are similar. Find the distance "d" across the Red River

Answer 1

The distance across the Red River (d) is 1.62 km.

In similar triangles, corresponding sides are proportional. Let's denote the sides of the two triangles as follows:

Triangle 1:

a,b,c (where c is the side across the Red River)

Triangle 2:

A,B,C (where C is the corresponding side in Triangle 2)

Given that the triangles are similar, you can set up the following proportion:

`(a)/(A) =(b)/(B) =(c)/(C)`

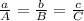
In your case, you mentioned that the sides of Triangle 1 are 0.9 km, 1 km, and d km, and the sides of Triangle 2 are 0.9 km, 1.8 km, and an unknown side (let's call it D km).

Setting up the proportion:

`(0.9)/(0.9) = (1)/(1.8) =(d)/(D)`

Now, you can solve for d by cross-multiplying:

0.9×1.8=1×d

1.62=d

So, the distance across the Red River (d) is 1.62 km.

Answer 2

2 km... Since 1.8 is 2(.9), you do the same for the base of the smaller triangle (just multiply by two)