Question

Determine the number of real solutions each quadratic equation has. y = 12x2 - 9x 4

Answer 1

Find the Vertex of y = 12x2-9x+4
y = 2.313

Answer 2

ANSWER

The quadratic function,


`y = 12 {x}^(2) - 9x + 4`
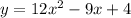
has no real roots.


Step-by-step explanation

We use the discriminant,


`D = {b}^(2) - 4ac`

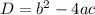
to determine the nature of the roots of a quadratic equation.


If we compare


`y = 12 {x}^(2) - 9x + 4`

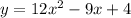
to


`y = a{x}^(2) + bx + c`



Then


`a=12,b=-9,c=4`


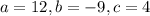
We substitute these values in to the formula for the discriminant, to obtain,




`D = {( - 9)}^(2) - 4(12)(4)`



`D = 81 - 192`


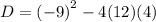
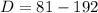
This implies that,


`D = - 111 \: < \: 0`


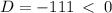
Since the discriminant is zero, the quadratic function,



`y = 12 {x}^(2) - 9x + 4`

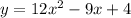
has no real roots.