Question

An airplane leaves an airport and flies due west 150 miles and then 170 miles in the direction S 49.17°W. How far is the plane from the airport (round to the nearest mile)?

Answer 1

notice the picture below

what we have, is really an angle, encroached by two sides

thus, use the Law of Cosines


`\bf \textit{Law of Cosines}\\ \quad \\ c^2 = {{ a}}^2+{{ b}}^2-(2{{ a}}{{ b}})cos(C)\implies c = \sqrt{{{ a}}^2+{{ b}}^2-(2{{ a}}{{ b}})cos(C)}\\\\ -----------------------------\\\\ \textit{so the distance`

Answer 2

The distance from plane to airport is 134 miles.

Explanation:

Given an airplane leaves an airport and flies due west 150 miles and then 170 miles in the direction S 49.17°W. we have to find the distance of plane from the airport.

Given the sides c and a that are

c=150 miles and a=170 miles also ∠B=49.17°

we have to calculate b

By law of cosines,

`b^2=a^2+c^2-2ac\thinspace cosB`

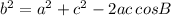
Substitute the values, we get

`b^2=(170)^2+(150)^2-2*170*150cos(49.17^(\circ))`

⇒
`b^2=51400-51000cos(49.17^(\circ))`

⇒
`b=134.370157846\sim134miles`

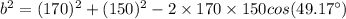
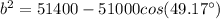
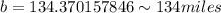
The distance from plane to airport is 134 miles.