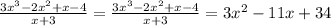Question

Use synthetic division to find the quotient. If there is a remainder do not include it in your answer. Recall:dividend\div divisor=quotient (3x^3-2x^2+x-4) \div(x+3) Be sure to type your answer in descending powers of x with now spaces between your terms. Use the "^" key (shift+6) to indicate a power/exponent.Answer:

Answer 1

`3x^2-11x+34`

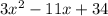
Explanation:

Given the division below:

`(3x^3-2x^2+x-4)/(x+3)`

First, set the denominator equal to 0 and solve for x.

`\begin{gathered} x+3=0 \\ x=-3 \end{gathered}`

Next, set the synthetic division table as shown below:

• Bring down the leading coefficient, 3.

,

• Then multiply 3 by -3, write the result in the next column and add.

Repeat the process until you get the sum of the last column.

Therefore:

`(3x^3-2x^2+x-4)/(x+3)=3x^2-11x+34-(106)/(x+3)`

Following the instruction in the question, we ignore the remainder and write:

`(3x^3-2x^2+x-4)/(x+3)=(3x^3-2x^2+x-4)/(x+3)=3x^2-11x+34`