Question

Blank High School sells Prom tickets for $75 per couple and $50 per single. If a total of 173 students attended the prom and blank High School earned $7050. How many of each type of ticket did blank High School sell.

Answer 1

Let x be the number of couple tickets and let y be the number of single tickets sold.

We know that the school earned a total of $7050, then we have that:

`75x+50y=7050`

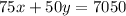
Now, we also know that the total number of students was 173, then we have:

`2x+y=173`

Hence we have the system of equations:

`\begin{gathered} 75x+50y=7050 \\ 2x+y=173 \end{gathered}`

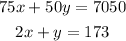
To solve this system we multiply the second equation by -50, then we have:

`\begin{gathered} 75x+50y=7050 \\ -100x-50y=-8650 \end{gathered}`

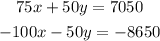
Adding this equation we have:

`\begin{gathered} -25x=-1600 \\ x=(-1600)/(-25) \\ x=64 \end{gathered}`

Once we know the value of x we plug it in the second equation to get y:

`\begin{gathered} 2(64)+y=173 \\ y=173-128 \\ y=45 \end{gathered}`

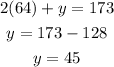
Therefore, they sold 64 couple tickets and 45 single tickets.