Answer:
(a) From the graph we get that the points (100, 30 000) and (200, 30 000) are on the parabola, therefore the largest possible value of x such that the are is 30 000 m² is
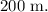
(b) To find the largest possible area we calculate the vertex of the parabola.
Taking the given equation to its standard form we get:
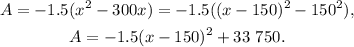
Therefore, the coordinates of the vertex are:
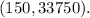
From the vertex we get that, the value of x that maximizes the area is x=150.
Substituting x=150 in 3x+2y=900, and solving for y, we get:
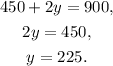
The values of x and y that maximize the area are x=150m, y=225 m.
(c) From the previous step we get that the maximum area of the paddock is 33 750 m².