Question

State where the function is continuous, discontuous and the type of discontinuity for each

Answer 1

`f(x)=\begin{cases}(x)/(x-2);x<2 \\ 6;2\leq x\leq6 \\ (x-2)/(x^2-6x+8);x>6\end{cases}`

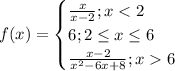
For x = 2

`\lim _(x->2^-)((x)/(x-2))=-\infty`
`\begin{gathered} \lim _(x->2^+)(6)=6 \\ \end{gathered}`

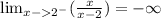
Since:

`\lim _(x->2^+)f(x)\\e\lim _(x->2^-)f(x)`

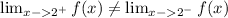
The function is discontinuous at x = 2. Besides since the function has a vertical asymptote on one of the sides, we can conclude it is a infinite discontinuity.

For x = 6:

`\lim _(x->6^-)6=6`
`\lim _(x->6^+)(x-2)/(x^2-6x+8)=\lim _(x->6^+)(x-2)/((x-2)(x-4))=\lim _(x->6^+)(1)/(x-4)=(1)/(2)`

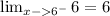
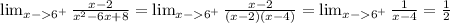
Since:

`\lim _(x->6^+)f(x)\\e\lim _(x->6^-)f(x)`

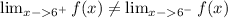
The function is discontinuous at x = 6, at this point the function has a jump discontinuity