Question

4. There are 130 students in grade one, 210 students in grade two and 290 students in grade three in a school, and so on in an arithmetic sequence. What’s the total amount of students in the school , if the school goes to grade 12?

Answer 1

Remember that the formula for an arithmetic sequence is

`a=dn+c`

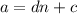
Where a is the term you want to find, d is the common difference, n is the number of the step you want to annalyse, and c is the value of the first term of the sequence.

In grade one (step zero of the sequence) we have 130 students, so

`c=130`

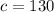
If we take the number of students in grade two (step one) and substract the 130 students in grade one, we can find out what the common difference is

`d=210-130\rightarrow d=80`

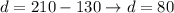
The formula of the arithmetic sequence would be

`a=80n+130`

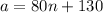
Notice it checks out with the number of students in grade three (step 2)

`a=80(2)+130\rightarrow a=290`

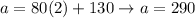
To know the total amount of students in the school we will have to add every single step of the sequence (from 0 to 11)

`\begin{gathered} 80(0)+130+80(1)+130+80(2)+130+80(3)+130+80(4)+130+80(5)+130 \\ +80(6)+130+80(7)+130+80(8)+130+80(9)+130+80(10)+130+80(11)+130 \end{gathered}`

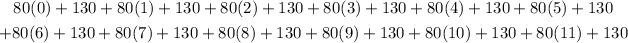
In other words,

`80(0+1+2+3+4+5+6+7+8+9+10+11)+130(12)=6840`

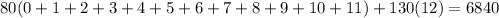
So, the total amount of students in the school , if the school goes to grade 12, would be 6840 students.