Question

The length of a rectangle is 8 inches less than 3 times the width. The perimeter is 88 inches. Find the length and the width.The length is ? inches and the width is ? inches.

Answer 1

The length is 35 inches

The width is 9 inches

Solution

- This question leads to a simultaneous equation with the length and width as variables.

- For the first equation, we simply need to interpret the logic in the question.

- For the second equation, we should apply the formula for the perimeter of a rectangle since we have been given the perimeter of the rectangle to be 88 inches.

- Thus, we can solve the question as follows:

First Equation

`\begin{gathered} The\text{ length of a rectangle is 8 inches less than 3 times the width} \\ 3\text{ times the width (w) is: 3}* w=3w \\ \\ \text{If the length of the rectangle is 8 inches less than 3 times the width or }3w,\text{ then it means that} \\ \text{when we subtract the length from }3w,\text{ we should get 8 inches} \\ \\ \text{If the length of the rectangle is }l\text{, then we can say:} \\ 3w-l=8\text{ (Equation 1)} \end{gathered}`

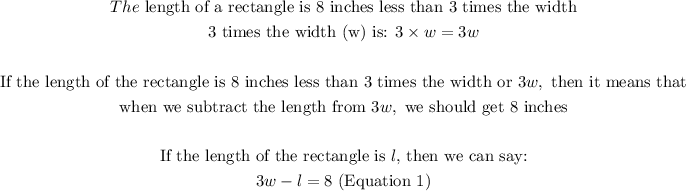
Second Equation

- Now, let us apply the formula for the perimeter of a rectangle to find the second equation

`\begin{gathered} P=2(l+w) \\ P=88 \\ 88=2(l+w) \\ \text{Divide both sides by 2} \\ (88)/(2)=(2(l+w))/(2) \\ \\ \therefore l+w=44\text{ (Equation 2)} \end{gathered}`

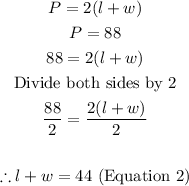
- Now, let us solve the equations simultaneously.

- We can use the substitution method as follows:

`\begin{gathered} 3w-l=8\text{ (Equation 1)} \\ l+w=44\text{ (Equation 2)} \\ \\ \text{Make }l\text{ the subject of the formula in equation 1} \\ l=3w-8 \\ \text{Substitute this expression for }l\text{ into equation 2.} \\ 3w-8+w=44 \\ \text{Collect like terms} \\ 4w=36 \\ \text{Divide both sides by 4} \\ (4w)/(4)=(36)/(4) \\ \\ \therefore w=9 \\ \\ \text{Let us substitute the value of }w\text{ into equation 2} \\ l+9=44 \\ \text{Subtract 9 from both sides} \\ l=44-9 \\ \therefore l=35 \end{gathered}`

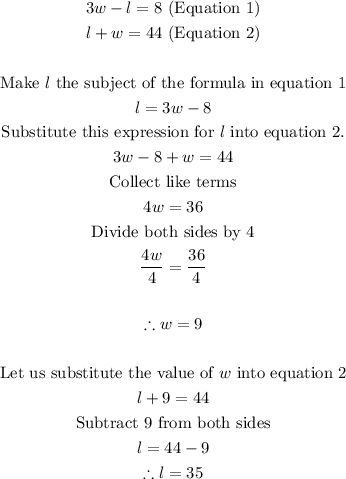
Final Answer

The length is 35 inches

The width is 9 inches