Question

In the following figure, triangle ABC is a right triangle, and m A = 42°. Find the value of n°. Note: the figure is not drawn to scale.

n =

Answer 1

42+90 = 132

2 non adjacent angles is equal to the exterior angle of a triangle.

Answer 2

`m\angle n=132^(\circ)`

Explanation:

We have been given an image of a right triangle and we are asked to find the measure of n.

We can see from given triangle that angle n is exterior angle of triangle ABC.

The exterior angle of triangle theorem states that the measure of an exterior angle of a triangle is equal to the sum of the measures of the two non-adjacent interior angles of the triangle.

Using above theorem we can set an equation to solve for n as:

`m\angle n=m\angle A+m\angle B`

`m\angle n=42^(\circ)+90^(\circ)`

`m\angle n=132^(\circ)`

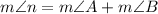
Therefore, the value of n is 132 degrees.