Question

Luke, Kira, and Ali each served 23 of their own cake. Each cake was the same size, but Luke served 4 slices, Kira served 6 slices, and Ali served 8 slices. How is this possible? Enter your answers in the boxes to complete the solution

Answer 1

Complete Question:

Luke, Kira, and Ali each served 2/3 of their own cake. Each cake was the same size, but Luke served 4 slices, Kira served 6 slices, and Ali served 8 slices. How is this possible? Enter your answers in the boxes to complete the solution.

A. Luke cut his cake into how many slices?

B. Kira cut her cake into how many slices?

C. Ali cut her cake into how many slices.

Answer:

A. 6 slices.

B. 9 slices.

C. 12 slices.

Explanation:

Given the following data;

Number served by Luke = 4 slices

Number served by Kira = 6 slices

Number served by Ali = 8 slices

We would solve for the number of slices each of them divided their cakes into.

For Luke, serving 2/3;

`\frac {4}{(2)/(3)} = \frac {4 * 3}{2}`

`\frac {4}{(2)/(3)} = \frac {12}{2}`

`\frac {4}{(2)/(3)} = 6 \; slices`

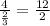
For Kira, serving 2/3;

`\frac {6}{(2)/(3)} = \frac {6 * 3}{2}`

`\frac {6}{(2)/(3)} = \frac {18}{2}`

`\frac {6}{(2)/(3)} = 9 \; slices`

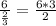
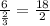
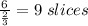
For Ali, serving 2/3;

`\frac {8}{(2)/(3)} = \frac {8 * 3}{2}`

`\frac {8}{(2)/(3)} = \frac {24}{2}`

`\frac {8}{(2)/(3)} = 12 \; slices`

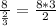
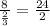
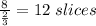
Therefore, it is possible because;

A. Luke cut his cake into 6 slices.

B. Kira cut her cake into 9 slices.

C. Ali cut her cake into 12 slices.