Question

Find the perimeter of a quadrilateral with vertices at C (−2, 1), D (2, 4), E (5, 0), and F (1, −3). a12 units b16 units c20 units d24 units

Answer 1

20

Explanation:

Use the distance formula to find the length of all the sides, then add each length. or use the formula for perimeter.

Answer 2

To find the perimeter of a quadrilateral, we need to add the lengths of the sides. For this, we use the distance formula:

`d=√((x_1-x_2)^2+(y_1-y_2)^2)`

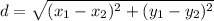
Let's solve for each of the sides.

`\begin{gathered} CD=√((-2-2)^2+(1-4)^2) \\ \\ CD=√(16+9) \\ \\ CD=√(25)=5 \end{gathered}`
`\begin{gathered} DE=√((2-5)^2+(4-0)^2) \\ DE=√(9+16) \\ DE=√(25)=5 \end{gathered}`
`\begin{gathered} EF=√((5-1)^2+(0-(-3))^2) \\ EF=√(16+9) \\ EF=√(25)=5 \end{gathered}`
`\begin{gathered} FC=√((1-(-2))^2+(-3-1)^2) \\ FC=√(9+16) \\ FC=√(25)=5 \end{gathered}`

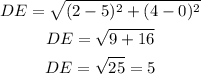
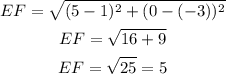
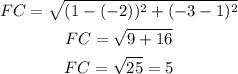
So each of the sides of the quadrilateral measures 5 units.

The perimiter is the sum of all the sides. P =5 + 5 + 5 + 5 = 4 x 5 = 20 units.