Question

Fredo deposits $75 a year in an account earning 3% interest compounded annual. if he deposits $75 a year and does not make any withdrawals, how much interest will the account earn in the fourth year?

Answer 1

Total = principal * (1+rate)^years

Total = 75 * (1.03)^4 = 84.41 = 9.41 Interest
Total = 75 * (1.03)^3 = 81.95 = 6.95 Interest
Total = 75 * (1.03)^2 = 79.57 = 4.57 Interest
Total = 75 * (1.03)^1 = 77.25 = 2.25 Interest

Four Years of Interest = $23.18

Answer 2

The interest the account will earn in the fourth year is:

$ 9.4132

Explanation:

We know that the amount obtained on a account if the interest is compounded annually is given by:

`A=P(1+r)^t`

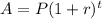
where P is the principal amount.

r is the rate at which the interest is calculated in decimal form.

t is the time i.e. number of years.

and A is the amount after t years.

Hence, the interest obtained is:

`\text{Interest obtained}=A-P`

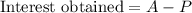
Here we are given :

P=$ 75

r= 0.03

and we are asked to calculate the interest when t= 4 .

Hence, we have:

`A=75(1+0.03)^4\\\\A=75(1.03)^4\\\\A=84.4132`

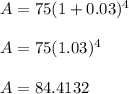
Hence,

`\text{Interest obtained}=84.4132-75\\\\\text{Interest obtained}=\$ 9.4132`