Answer:
Option B is correct
3 m
Explanation:
Using tangent ratio:
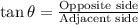
As per the statement:
Carlota has leaned a ladder against the side of her house.
The ladder forms a 53˚ angle with the ground and rests against the house at a spot that is 4 meters high
From the given diagram:
⇒Angle of elevation
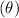 = 53 degree and BC = 4 m
= 53 degree and BC = 4 m
To find AB:
Using tangent ratio:
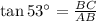
⇒
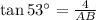
⇒
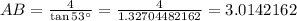 m
m
Therefore, the best approximation for the distance along the ground from the bottom of the ladder to the wall length is, 3 m