Question

The equation of a circle is x2+y2+8x−14y+56=0 .

What is the radius of the circle?


r =   units

Answer 1

Answer: The radius of the circle is 3 units.

Step-by-step explanation: The given equation of the circle is

`x^2+y^2+8x-14y+56=0~~~~~~~~~~~~~~~(i)`

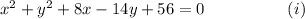
We are to find the radius of the circle (i).

The standard equation of a circle with centre (g, h) and radius 'r' units is given by

`(x-g)^2+(y-h)^2=r^2.`

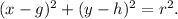
From equation (i), we have

`x^2+y^2+8x-14y+56=0\\\\\Rightarrow (x^2+8y+16)+(y^2-14y+49)-16-49+56=0\\\\\Rightarrow (x+4)^2+(y-7)^2=9\\\\\Rightarrow (x+4)^2+(y-7)^2=3^2.`

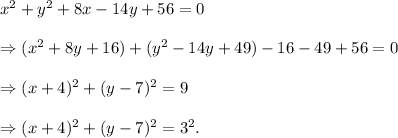
Comparing the above equation with the standard equation of a circle, we get

r = 3.

Thus, the radius of the given circle is 3 units.

Answer 2

the main formula of a circle is (x-a)²+(y-b)²= r², where (a,b) is the center of the circle
x2+y2+8x−14y+56= x2+8x+y2−14y+56=(x+4)²-16+(y-7)²- 49+ 56=0
it means (x+4)²+(y-7)²- 9=0 and it implies (x+4)²+(y-7)²=9
so r²=9, and finally r =3