There is a nice shortcut for long division of polynomials when dividing by divisors of the form
 . We name this shortcut as synthetic division.
. We name this shortcut as synthetic division.
- To divide
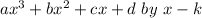 we use the pattern for synthetic division of a cubic polynomial summarized in Figure 1.
we use the pattern for synthetic division of a cubic polynomial summarized in Figure 1.
- You should set up the arrays as indicated in Figure 2.
- Then use the synthetic division pattern by adding terms in columns and multiplying the results by 3 as illustrated in Figure 3.
Finally, the quotient is:
