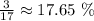NUMBER 5:
INFORMATION:
We have a trapeze and, we need to find the probability that a randomly chosen point is the figure lies in the shaded region
STEP BY STEP EXPLANATION:
To find the probability, we must divide the area of the shaded region by the total area of the trapeze

- Total area:
To calculate the total area, we must use the formula for the area of a trapeze

Where, b1 and b2 are the bases and h is the height
Then, analyzing the trapeze we can see that b1 = 20, b2 = 14 and h = 12
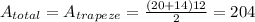
So, the total area is 204 square units
- Shaded area:
To find the shaded area, we must subtract the no shaded area from the total area.
We can see that the no shaded area is a rectangle with width = 14 and height = 12
Now, using the formula for the area of a rectangle
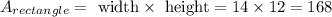
Then, subtracting the area of the rectangle from the total area
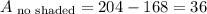
So, the no shaded are is 36 square units.
Finally, the probability would be
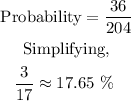
ANSWER:
the probability that a randomly chosen point is the figure lies in the shaded region is