Question

Malene wrote the equation x² + 8x + 60 = x² + 12x + 20 to show that the area of a changed rectangle is 60 cm² greater than the area of the original rectangle. How did the rectangle change?

1 was added to each side.
2 was added to each side.
8 was added to each side.
10 was added to each side.

Answer 1

The answer is 10 was added to each side.

x² + 8x + 60 = x² + 12x + 20

x² + 8x + 60 - x² = x² + 12x + 20 - x²

8x + 60 = 12x + 20
60 - 20 = 12x - 8x
40 = 4x
x = 40/4
x = 10

So, 10 was added to each side.

Answer 2

2 was added to each side

Explanation:

Given that a rectangle when changed sides, the area became 60 greater than the original

The equation given is

`x^2 + 8x + 60 = x^2 + 12x + 20`

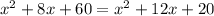
From the above equation we can see that the original area was
`x^2 + 8x`

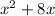
and it changed to

`x^2 + 12x + 20`

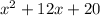
Original rectangle dimensions= factors of area terms = x , x+8

New rectangle dimensions = factors of
`x^2 + 12x + 20`

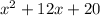
=x+2, x+10

Thus we have both length and width increased by 2 units.