Answer:
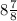
Step-by-step explanation:
It has been given that you want to place
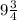 inch towel bar in the center of a door that is
inch towel bar in the center of a door that is
 wide.
wide.
First of all, we will find the total space left on both sides of towel bar by subtracting
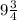 from
from
 .
.

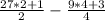


Let us have a common denominator.
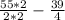
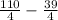
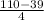
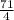
Now, we will divide
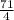 by 2 to find the space left on each side of the towel bar.
by 2 to find the space left on each side of the towel bar.

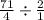
By flipping the second fraction and multiplying by 1st fraction we will get,
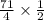
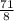
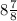
Therefore,
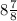 inch of space will be on each side of the towel bar.
inch of space will be on each side of the towel bar.