Question

Please Help !!!!

How many roots does the polynomial function y=(x-2)(x+3)^2 have ?
A. 2
B. 3
C. 1
D. 4

Answer 1

It is A. 2 because it is the same as the highest degree

Answer 2

Polynomial has three roots.

B is the correct option.

Explanation:

We have been given the function
`y=(x-2)(x+3)^2`

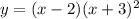
We know that the number of roots or zeros of a polynomial is equal to the degree of the polynomial.

Let us simplify the given function and find the degree.

`y=(x-2)(x+3)^2\\\\y=(x-2)(x^2+6x+9)\\\\y=x^3+6x^2+9x-2x^2-12x-18\\\\y=x^3+4x^2-3x-18`

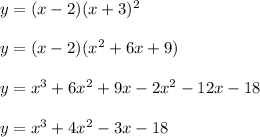
Since, the degree of the polynomial is 3.

Hence, it must have three zeros or roots.

B is the correct option.