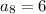Answer:
Explicit formula for the sequence is
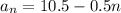 and
and
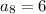
Explanation:
Given: Sequence = 10, 9.5 , 9 , 8.5 , 8
To find: Explicit Formula for the sequence and 8th term of sequence
1st term of sequence = 10
2nd term of sequence = 9.5
3rd term of sequence = 9
4th term of sequence = 8.5
5th term of sequence = 8
Difference between 2nd and 1st term = 9.5 - 10 = -0.5
Difference between 3rd and 2nd term = 9 - 9.5 = -0.5
Since, Difference is same in both cases
⇒ It is Arthematic Progression
⇒ First term, a = 10 and Common term, d = -0.5
using formula of AP for nth term we get,
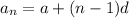
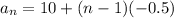
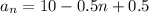
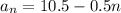
⇒ 8th Term of AP,
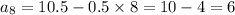
Therefore, Explicit formula for the sequence is
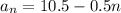 and
and