Question

How does the value of log2^100 compare with the value of log6^20

Answer 1

log_2(100) is about 4 times the value of log_6(20)

Explanation:

Lets find the value of both logs and then compare

`log_2(100)`

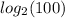
To find the value we use change of base formula

`log_b(a) = (log(a))/(log(b))`

`log_2(100) = (log(100))/(log(2))= 6.643856`

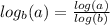
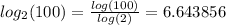
Now we find the value of log_6(20)

`log_6(20) = (log(20))/(log(6))=1.67195`

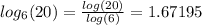
6.643556 is approximately four times of 1.67195

So log_2(100) is about 4 times the value of log_6(20)