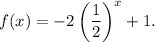Question

Given its parent function g(x) = (1/2)^x, what is the equation of the function shown?

Answer 1

so... notice, the graph is just g(x), flipped upside-down and shifted up by 1 unit

Answer 2

Answer: The correct equation is (B)
`f(x)=-2\left((1)/(2)\right)^x+1.`

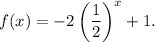
Step-by-step explanation: We are given to select the correct equation of the function f(x) shown in the figure.

From the graph given in the figure, we have

`f(-1)=-3,~~f(0)=-1,~~f(1)=0.`

(A) First option is

`f(x)=-3\left((1)/(2)\right)^x+1.`

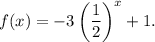
From here, we get

`f(-1)=-3\left((1)/(2)\right)^(-1)+1=-3* 2+1=-5\\eq -3.`

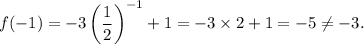
So, this option is not correct.

(B) First option is

`f(x)=--2\left((1)/(2)\right)^x+1.`

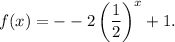
From here, we get

`f(-1)=-2\left((1)/(2)\right)^(-1)+1=-2* 2+1=-3\\\\f(0)=-2\left(1)/(2)\right)^0+1=-2+1=-1,\\\\f(1)=-2\left((1)/(2)\right)^1+1=-1+1=0.`

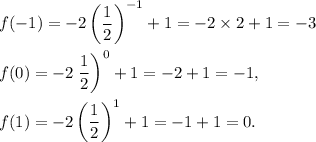
So, this option is correct.

(C) Third option is

`f(x)=-\left((1)/(2)\right)^x-3.`

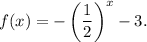
From here, we get

`f(-1)=-\left((1)/(2)\right)^(-1)-3=-1* 2-3=-5\\eq -3.`

So, this option is not correct.

(D) Fourth option is

`f(x)=-\left((1)/(2)\right)^x-1.`

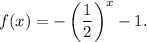
From here, we get

`f(-1)=-\left((1)/(2)\right)^(-1)-1=--* 2-1=-3,\\\\f(0)=-\left(1)/(2)\right)^0-1=-1-1=-2\\eq -1.`

So, this option is not correct.

Thus, the correct function is

(B)
`f(x)=-2\left((1)/(2)\right)^x+1.`