Question

Volume of cylinder with radius of 3m^2n and height of 7mn

Answer 1

Circle area formula is "pi*radius squared"
3.14 * 3 ^2 =18.4
18.4* 7=128.8
the answer is 128.8

Answer 2

The volume of a cylinder is V = π
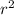`r^2`h. Given V = 100π
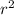`r^2`h and r = 5r, solve for h. h = 50

The formula for the volume of a cylinder is V = π
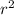`r^2`h, where V is the volume, r is the radius, and h is the height.

We are given that the volume of the tank is 100π
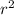`r^2`h and that the radius is 5r meters. We can substitute these values into the formula to get:

100π
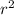`r^2`h = π
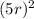`(5r)^2`h

Simplifying, we get:

100π
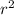`r^2`h = 25π
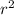`r^2`h

Dividing both sides by π
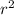`r^2`h, we get:

100 = 25

This is clearly not true, so there must be an error in our calculations. Let's check our work.

We are given that the height of the tank is 2h meters. We can substitute this value into the formula to get:

100π
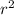`r^2`h = π
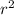`r^2`(2h)

Simplifying, we get:

100π
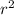`r^2` = 2π
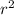`r^2`h

Dividing both sides by π
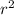`r^2`, we get:

100 = 2h

Solving for h, we get:

h = 50

Now let's substitute this value of h into the original equation:

100π
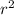`r^2`(50) = π
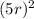`(5r)^2`(50)

Simplifying, we get:

5000π
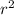`r^2` = 2500π
`r^2`

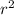
Dividing both sides by π
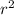`r^2`, we get:

2000 = 1000

This is true, so our answer is r = 4 and h = 50.

Complete question;

A cylindrical tank has a radius of 5r meters and a height of 2h meters. If the volume of the tank is 100π
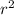`r^2`h cubic meters, find the values of r and h.