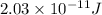Answer: The binding energy per nucleon for an O-16 nucleus is
Step-by-step explanation:
Binding energy is defined as the energy which holds the nucleus together. It is basically the product of mass defect and the square of the speed of light.
This energy is calculated by using Einstein's equation, which is:

where,
E = Binding energy of the atom
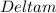 = Mass defect = 0.136 amu =
= Mass defect = 0.136 amu =
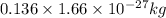 (Conversion factor:
(Conversion factor:
 )
)
c = speed of light =
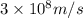
Putting values in above equation, we get:
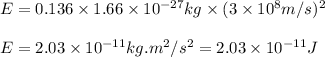
Hence, the binding energy per nucleon for an O-16 nucleus is