Answer:
Option d - $63,126.00
Explanation:
Given : You would like to withdraw a monthly salary of $1,205.78 from an account paying 5.5% interest, compounded monthly.
To find : Determine the amount needed in the account such that you can withdraw the needed amount at the end of each month for 5 years?
Solution :
Applying formula of monthly payment ,
Monthly payment,
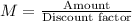
Discount factor
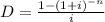
Where, Amount = ?
Monthly payment = $1205.78
Rate r= 5.5%=0.055
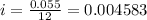
Time = 5 years
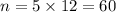
Now, put all the values we get,
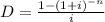
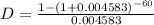
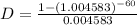
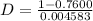
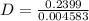
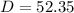
Substitute the value in the formula,
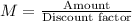


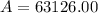
Therefore, Option d is correct.
The amount needed in the account is $63,126.00