Question

A 1.50-kilogram ball is attached to the end of a 0.520-meter string and swung in a circle. The velocity of the ball is 9.78 m/s. What is the tension in the string? 74.6 N 276 N 28.2 N 3.39 N

Answer 1

Data:
Tension = Centripetal Force = ? (Newton)
m (mass) = 1.50 Kg
s (speed) = 9.78 m/s
R (radius) = 0.520 m (The piece of this rope with the ball tied in circular motion forms the circular radius).

Formula:

`F_(centripetal\:force) = (m*s^2)/(R)`

Solving:

`F_(centripetal\:force) = (m*s^2)/(R)`

`F_(centripetal\:force) = (1.50*9.78^2)/(0.520)`

`F_(centripetal\:force) = (1.50*95.6484)/(0.520)`

`F_(centripetal\:force) = (143.4726)/(0.520)`

`F_(centripetal\:force) = 275.9088... \to\:\boxed{\boxed{F_(centripetal\:force) \approx 276\:N}}\end{array}}\qquad\quad\checkmark`

Answer:
The tension in the string is 276 N

Answer 2

The tension in the string is 276 N.

Step-by-step explanation:

It is given that,

Mass of the ball, m = 1.5 kg

Length of the meter stick, r = 0.52 m

Velocity of the ball, v = 9.78 m/s

The tension acting in the string is balanced by the centripetal force acting on it. Its formula is given by :

`F_c=(mv^2)/(r)`

`F_c=(1.5\ kg* (9.78\ m/s)^2)/(0.52\ m)`

`F_c=275.90\ N`

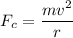
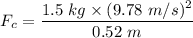
or

`F_c=276\ N`

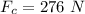
So, the tension in the string is 276 N. Hence, this is the required solution.