Answer:
The potential real zeros are
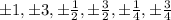 .
.
Explanation:
The given polynomial is
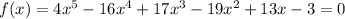
According to the rational root theorem, all the potential real zeros are in the form of
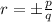
Where, p is the factor of constant and q is the factor of leading coefficient.
The constant term is -3 and leading coefficient is 4.
Factors of -3 are ±1, ±3.
Factors of 4 are ±1, ±2, ±4.
The potential real zeros are
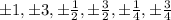
Therefore potential real zeros are
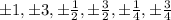 .
.