Question

What are the coordinates of the focus of the conic section shown below (y+2)^2/16-(x-3)^2/9=1

Answer 1

Answer :It's A lol

Explanation:

Answer 2

Given the function of the conic section:

`\mleft(y+2\mright)^2/16-\mleft(x-3\mright)^2/9=1`

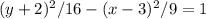
This conic section is a hyperbola.

Use this form below to determine the values used to find vertices and asymptotes of the hyperbola:

`((x-h)^2)/(a^2)\text{ - }((y-k)^2)/(b^2)\text{ = }1`

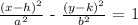
Match the values in this hyperbola to those of the standard form.

The variable h represents the x-offset from the origin b, k represents the y-offset from origin a.

We get,

a = 4

b = 3

k = 3

h = -2

A. The first focus of a hyperbola can be found by adding the distance of the center to a focus or c to h.

But first, let's determine the value of c. We will be using the formula below:

`\sqrt[]{a^2+b^2}`

Let's now determine the value of c.

`\sqrt[]{a^2+b^2}\text{ = }\sqrt[]{4^2+3^2}\text{ = }\sqrt[]{16\text{ + 9}}\text{ = }\sqrt[]{25}`
`\text{ c = 5}`

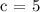
Let's now determine the coordinates of the first foci:

`\text{Coordinates of 1st Foci: (}h\text{ + c, k) = (-2 + 5, 3) = 3,3}`

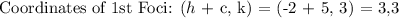
B. The second focus of a hyperbola can be found by subtracting c from h.

`\text{ Coordinates of 2nd Foci: (h - c, k) = (-2 - 5, 3) = -7,3}`

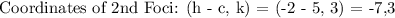
Therefore, the conic section has two focus and their coordinates are 3,3 and -7,3.

In other forms, the foci of the hyperbola is:

`\text{ }(h\text{ }\pm\text{ }\sqrt[]{a^2+b^2},\text{ k) or (-2 }\pm\text{ 5, 3)}`

Therefore, the answer is letter B.