Answer:
F' = 162 units
Step-by-step explanation:
The gravitational force of attraction between the two objects is given by Newton's Gravitational law through the following formula:

where,
F = gravitational force = 18 units
G = Gravitational Constant
m₁ = mass of object 1
m₂ = mass of object 2
r = distance between objects
Therefore,
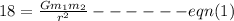
Now, if we change the value of distance to one-third of original value, then:
r' = r/3

using eqn (1):
F' = 9(18 units)
F' = 162 units