ANSWER
y = -0.25 + 7
Step-by-step explanation
The line passes through the points (-4, 8) and (12, 4).
The slope-intercept form of a linear equation is written as:
y = mx + c
where m = slope
c = y intercept
First, we have to find the slope of the line.
We do that with formula:

Therefore, the slope is:
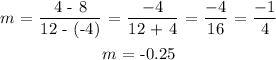
Now, we use the point-slope method to find the equation:
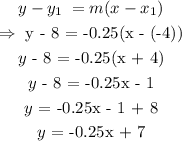
That is the equation of the line. It is not among the options.