Step-by-step explanation:
We know that there is a relation between the wavelength and the frequency of wave. It is given by :
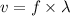
Where
v is the speed of a wave
f is the frequency
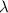 is the wavelength
is the wavelength
Let T is the wave period of a wave. The frequency of a wave is inversely proportional to the time period. Its formula is given by :
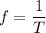
When the wave period of the wave increases, its frequency gets decreased. As a result, the speed of wave decreases.