Question

I got first part correct but dont know how to solve second part: Two new particles with identical positive charge 3 are placed the same 0.0809 m apart. The force between them is measured to be the same as that between the original particles. What is 3 ?

Answer 1

5.92 *10^-6 C

Step-by-step explanation:

For the two charges q3 the force between them is given by

`F=k(q_3* q_3)/(d^2)`

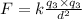
Now we know that

F = 48.1 N, d = 0.0809 m, and k = 8.99 *10^9 kg⋅m^3⋅s^−2⋅C^-2; therefore, the above gives

`48.1=(8.99*10^9)(q_3* q_3)/((0.0809)^2)`
`\Rightarrow48.1=(8.99*10^9)((q_3)^2)/((0.0809)^2)`

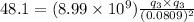
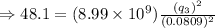
Now we solve for q_3.

Dividing both sides by 8.99 * 10^9 gives

`(48.1)/((8.99*10^9))=((q_3)^2)/((0.0809)^2)`

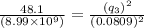
multiplying both sides by (0.0809)^2 gives

`(48.1)/((8.99*10^9))*\mleft(0.0809\mright)^2=(q_3)^2`

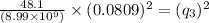
finally, taking the square root of both sides gives

`\sqrt[]{(48.1)/((8.99*10^9))*(0.0809)^2}=√((q_3)^2)`
`q_3=\sqrt[]{(48.1)/((8.99*10^9))*(0.0809)^2}`

Evaluating the right-hand side gives

`\boxed{q_3=_{}5.92*10^(-6)C\text{.}}`

Hence, the charge q_3 is 5.92 x 10^-6 C.