Question

Multiple-Choice Integration, Picture Included, Please Include Work

Answer 1

`\displaystyle\int_0^2√(4-x^2)\,\mathrm dx`

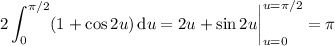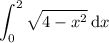
Recall that a circle of radius 2 centered at the origin has equation


`x^2+y^2=4\implies y=\pm√(4-x^2)`

where the positive root gives the top half of the circle in the x-y plane. The definite integral corresponds to the area of the right half of this top half. Since the area of a circle with radius
`r` is
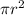
`\pi r^2`, it follows that the area of a quarter-circle would be
`\frac{\pi r^2}4`.

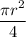
You have
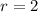
`r=2`, so the definite integral is equal to
`\frac{2^2\pi}4=\pi`.

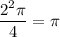
Another way to verify this is to actually compute the integral. Let
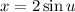
`x=2\sin u`, so that
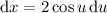
`\mathrm dx=2\cos u\,\mathrm du`. Now


`\displaystyle\int_0^2√(4-x^2)\,\mathrm dx=\int_0^(\pi/2)√(4-(2\sin u)^2)(2\cos u)\,\mathrm du=4\int_0^(\pi/2)\cos^2u\,\mathrm du`

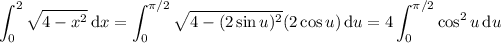
Recall the half-angle identity for cosine:


`\cos^2u=\frac{1+\cos2u}2`

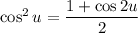
This means the integral is equivalent to


`\displaystyle2\int_0^(\pi/2)(1+\cos 2u)\,\mathrm du=2u+\sin2u\bigg|_(u=0)^(u=\pi/2)=\pi`