By the rational root theorem, you have the following candidates for roots:
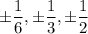
Plugging in each of these will tell you which one is actually a zero. You'll find that both
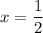
and
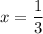
both work, which means
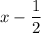
and

are linear factors to the quartic.
To find the remaining factor(s), divide the quartic by the known factors:
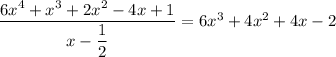
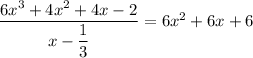
Since
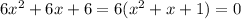
has no real roots, you are left with
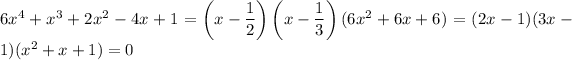
which has two real zeros at
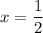
,
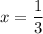
. It also has two complex roots at

.