Question

An investment of $6,599.20 earns 4.2% interest compounded monthly over 7 years. Approximately how much interest is earned on the investment?

Answer 1

Fv=6599.20(1+0.042/12)^12*7
Fv=8850.20
Interest earned=8850.20-6599.20
=2,251
So the answer is a

Answer 2

Find out the how much interest is earned on the investment .

To prove

Formula

`Amount =P ( 1 +(r)/(12))^(12t)`

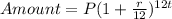
Where P is the principle, r is rate in decimal form , t is the time.

As given

An investment of $6,599.20 earns 4.2% interest compounded monthly over 7 years.

4.2% is written in the decimal form

`= (4.2)/(100)`

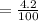
= 0.042

P = $6,599.20

T = 7 years

Put in the formula

`Amount = 6599.20( 1 +(0.042)/(12))^(12* 7)`

`Amount = 6599.20( 1 +(0.042)/(12))^(84)`

`Amount = 6599.20( 1 + 0.0035)^(84)`

`Amount = 6599.20( 1.0035)^(84)`

`Amount = 6599.20* 1.3411`

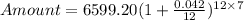
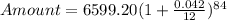
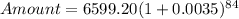
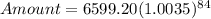
Amount = $ 8850.187

Interest = Amount - Principle

= $ 8850.187 - $6,599.20

= $2250.987

Therefore the interest is $2250.987

Option (a) is correct.