Question

The sum of two numbers is 83. The difference of the 2 numbers is 13. What is the product of the two numbers?A.1632B.1650C.1666D.1680

Answer 1

Let the first number be

`=x`

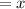
Let the second number be

`=y`

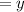
The sum of two numbers is 83 can be represented below as

`x+y=83\ldots\ldots(1)`

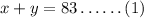
The difference of the 2 numbers is 13 can be represented below as

`x-y=13\ldots\ldots\text{.}(2)`

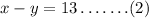
Step 1:

From equation (1) make x the subject of the formula to to give equation (3)

`\begin{gathered} x+y=83\ldots\ldots(1) \\ x=83-y\ldots\text{.}(3) \end{gathered}`

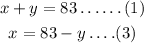
Step 2:

Substitute equation (3) in equation (2)

`\begin{gathered} x-y=13\ldots\ldots\text{.}(2) \\ x=83-y\ldots\text{.}(3) \\ 83-y-y=13 \\ 83-2y=13 \\ \text{collect similar terms,} \\ -2y=13-83 \\ -2y=-70 \\ \text{divide both sides by -2} \\ (-2y)/(-2)=(-70)/(-2) \\ y=35 \end{gathered}`

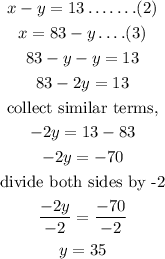
Step 3:

Substitute y= 35 in equation (3)

`\begin{gathered} x=83-y\ldots\text{.}(3) \\ x=83-35 \\ x=48 \end{gathered}`

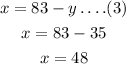
Hence,

The product of the two numbers will be calculated as

`\begin{gathered} =x* y \\ =35*48 \\ =1680 \end{gathered}`

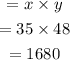
Hence,

The final answer is = 1680

OPTION D is the final answer