Question

A brass rod 100 mm long and 5 mm in diameter extends horizontally from a casting at 200°e. the rod is in an air environment with t∞ = 20°c and h = 30 w/m2 ∙ k. what is the temperature of the rod 25, 50, and 100 mm from the casting?

Answer 1

156°C, 128°C, and 106.5°C

Step-by-step explanation:

The temperature distribution can be calculated by the expression:

`(T)/(Tb) = (cosh m(L-x) + (h/mk)sinh m(L-x))/(coshmL + (h/mk)sinhmL)`

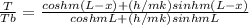
Where, L is the length (100 mm = 0.1 m), x is the distance to the end of the rod, k is the conductivity of the brass which is 133 W/m.K, and m is:

m =
`((hp)/(kAc))^(1/2)`

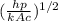
The perimeter, p = π*D, where D is the diameter (5 mm = 0.005m), and the area, Ac = π*D²/4

m =
`((4h)/(kD))^(1/2)`

m = 13.43 m⁻¹

So:

coshmL = cosh(13.43*0.1) = 2.05

sinhmL = sinh(13.43*0.1) = 1.78

h/mk = 30/(13.43*133) = 0.0168

Tb is the difference of temperature of the casting and the surroundings: Tb = 200 - 20 = 180°C

Thus,

T =
`180*((coshm(L-x) + 0.0168sinhm(L-x))/(2.05 + 0.0168*1.78) )`

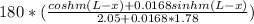
So, substituing the values of x:

x= 25 mm = 0.025 m

T =
`180*((cosh13.43(0.1 -0.025) + 0.0168sinh13.43(0.1-0.025))/(2.08) )`

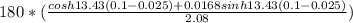
T = 136°C

T = Trod - T∞

Trod = 136 + 20

Trod = 156°C

x = 50 mm = 0.05 m

T =
`180*((cosh13.43(0.1 -0.05) + 0.0168sinh13.43(0.1-0.05))/(2.08) )`

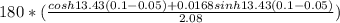
T = 108°C

Trod = 128°C

x = 100 mm = 0.1 m

T =
`180*((cosh13.43(0) + 0.0168sinh13.43(0))/(2.08) )`

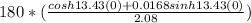
T = 86.5°C

Trod = 106.5°C