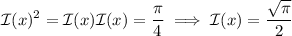Since
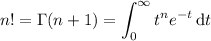
you have
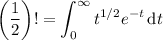
Let

, so that
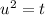
and
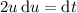
. This means the integral is equivalent to
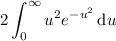
Integrating by parts, setting

,
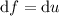
and
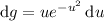
,
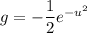
yields

You might already know that the value of this integral is
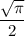
, so you're done. Or, if you're not familiar, you can derive this result.
If
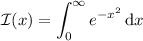
, then you have
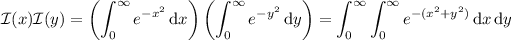
Converting to polar coordinates yields
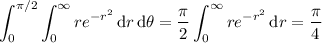
and so